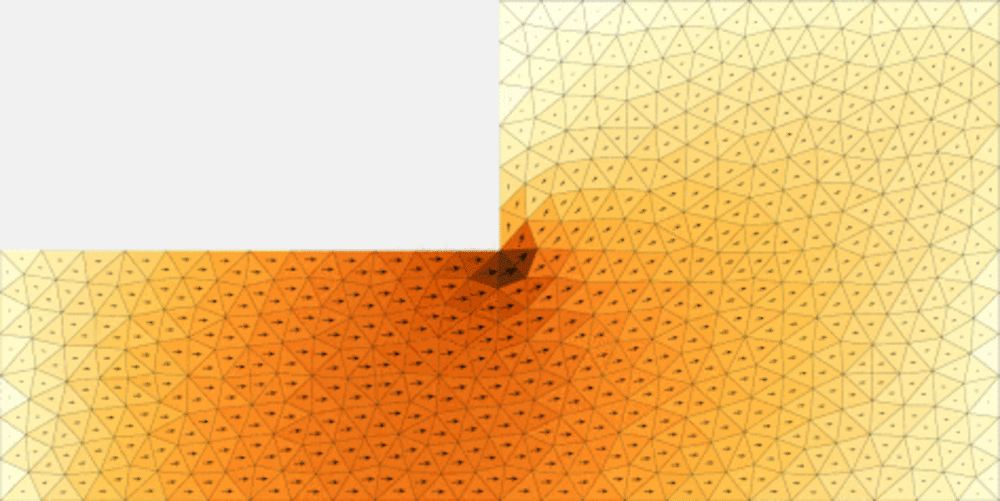
Fundamental bounds determine the best attainable values of physical metrics and are typically evaluated using tools of convex optimization and matrix operators. The fundamental bounds delimit performance of a hypothetical device. In line with the “source concept”, fundamental bounds result in optimal current densities defined in a prescribed region. Formulation of fundamental bounds commonly include physically motivated constraints such as enforcement of self-resonance or complex power balance. Among others, bounds are for example known on minimum antenna Q-factor, maximum antenna gain, minimum dissipation factor, minimum or maximum scattering cross sections.
Quite a few constraints can be used, e.g., a constraint on self-resonance, on complex power balance, or on only partly controllable region. All bounds can be transformed into multi-criteria form describing the mutual trade-offs.
Figure: Optimal current densities for an L-shape plate (electric size ka = 1/2). The sequence corresponds to a specific trade-off point between dissipation factor and Q-factor, maximal radiation efficiency (externally tuned and self-resonant), and minimal Q-factor, respectively.