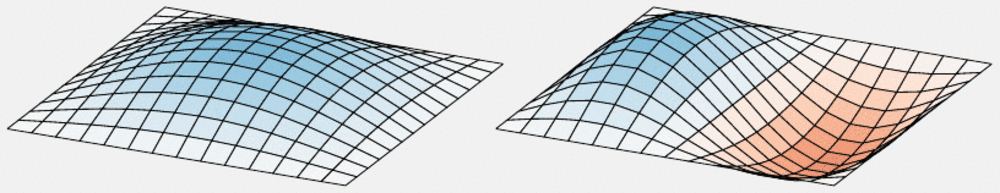
The most salient features of many complicated EM phenomena can be revealed by proper modal decomposition, which also reduces the complexity of problem and often offers additional physical insight. Most commonly, the modal decomposition is achieved via eigenvalue problem the modes of which are used as a new basis for the engineering problem at hand.
For example, characteristic modes diagonalize the impedance matrix, result in orthogonal far-fields, and are thus excellent for a design of electrically small MIMO antennas. Other bases, like radiation modes, are perfect for reducing the numerical complexity of fundamental bounds evaluation via convex optimization routines.
Figure: First two dominant modes on a rectangular plate.